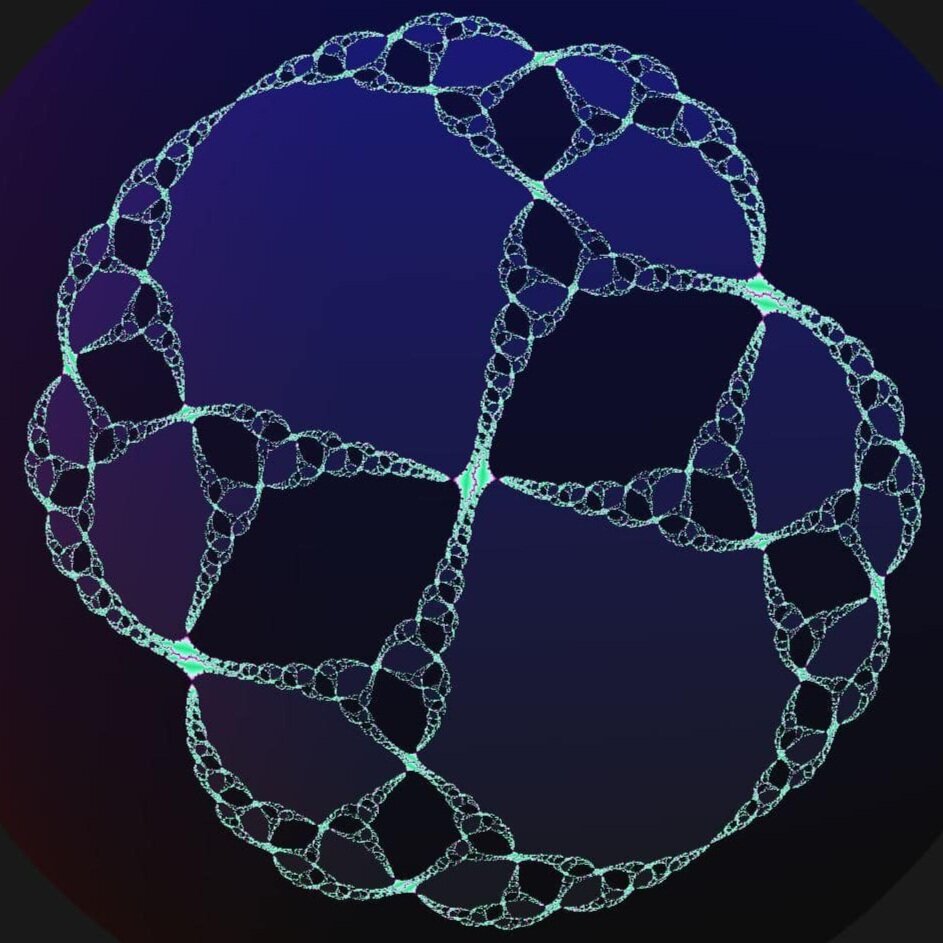
Project: Visualizing Julia Set Matings

Feb 27

Click to see gallery of full-sized images…

Introduction

While working on my Riemann Sphere animations, I happened across this work of Arnaud Chéritat which contained several videos of Julia Sets “mating” with one another. This instantly became of great interest to me, and promptly looked for an algorithm I could copy-paste into my already existing Riemann Sphere code. Unfortunately, the math behind polynomial matings was too complex for me to understand, and all the papers I read proved too dense for me to comprehend. Thankfully, I was able to find an answer by immersing myself in these snippets of code, which I discovered by asking this question. Using that algorithm, I created this simple project to be able to visualize Julia Set matings in real-time. A more expansive gallery of such animations can be found here.